8.4 Right Triangles and Pythagorus of Samos
Applications
Here’s the secret: We use right triangles all the time in forestry, wildlife, and water science – not because they are constantly found in nature, but because they have special mathematical properties that allow us to do amazing things with them. So, we build right triangles from the world around us to calculate measurements that might otherwise be inaccessible.

The Pythagorean Theorem
In a right triangle, the two sides that form the right angle are called the legs. The side opposite the right angle, which will always be the longest side, is called the hypotenuse.
The Pythagorean theorem says that the sum of the squares of the lengths of the legs is equal to the square of the length of the hypotenuse.

The Pythagorean Theorem
In a right triangle with legs [latex]a[/latex] and [latex]b[/latex] and hypotenuse [latex]c[/latex],
[latex]a^2+b^2=c^2[/latex]
If you know the lengths of all three sides of a triangle, you can use the Pythagorean theorem to verify whether the triangle is a right triangle or not. The ancient Egyptians used this method for surveying when they needed to redraw boundaries after the yearly flooding of the Nile washed away their previous markings.
The surveyors were called “rope-stretchers” because they used a loop of rope [latex]12[/latex] units long with [latex]12[/latex] equally-spaced knots. Three rope-stretchers each held a knot, forming a triangle with lengths [latex]3[/latex], [latex]4[/latex], and [latex]5[/latex] units. When the rope was stretched tight, they knew that the angle between the [latex]3[/latex]-unit and [latex]4[/latex]-unit sides was a right angle because [latex]3^2+4^2=5^2[/latex]. (From Discovering Geometry: an Inductive Approach by Michael Serra, Key Curriculum Press, 1997.)
Practice Exercises
Use the Pythagorean theorem to determine whether either of the following triangles is a right triangle.
Before we continue, we need to briefly discuss square roots. Calculating a square root is the opposite of squaring a number. For example, [latex]\sqrt{49}=7[/latex] because [latex]7^2=49[/latex]. If the number under the square root symbol is not a perfect square like [latex]49[/latex], then the square root will be an irrational decimal that we will round off as necessary.
Practice Exercises
Find the value of each square root. Round to the hundredths place.
- [latex]\sqrt{50}[/latex]
- [latex]\sqrt{296}[/latex]
- [latex]\sqrt{943}[/latex]
We most often use the Pythagorean theorem to calculate the length of a missing side of a right triangle. Here are three different versions of the Pythagorean theorem arranged to find a missing side, so you don’t have to use algebra with [latex]a^2+b^2=c^2[/latex].
The Pythagorean Theorem, three other versions
[latex]c=\sqrt{a^2+b^2}[/latex]
[latex]b=\sqrt{c^2-a^2}[/latex]
[latex]a=\sqrt{c^2-b^2}[/latex]
Examples: Using the Pythagorean Theorem
Practice Exercises
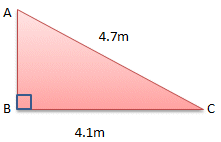
Find the length of the missing side for each of these right triangles. Round to the nearest tenth, if necessary.
View Practice Exercise Answers
Problem Set 8.4




![Pythagoras Theorem Questions [Solved]](https://cdn1.byjus.com/wp-content/uploads/2022/08/pythagoras-theorem-question-8.png)







